Equation Of A Circle General Form
Interactive coordinate geometry applet.
Equation of a circle general form. A circle is a simple closed shape. It is the set of all points in a plane that are at a given distance from a given point the centre. Equivalently it is the curve. The equation of a circle c is x plus 3 squared plus y minus 4 squared is equal to 49.
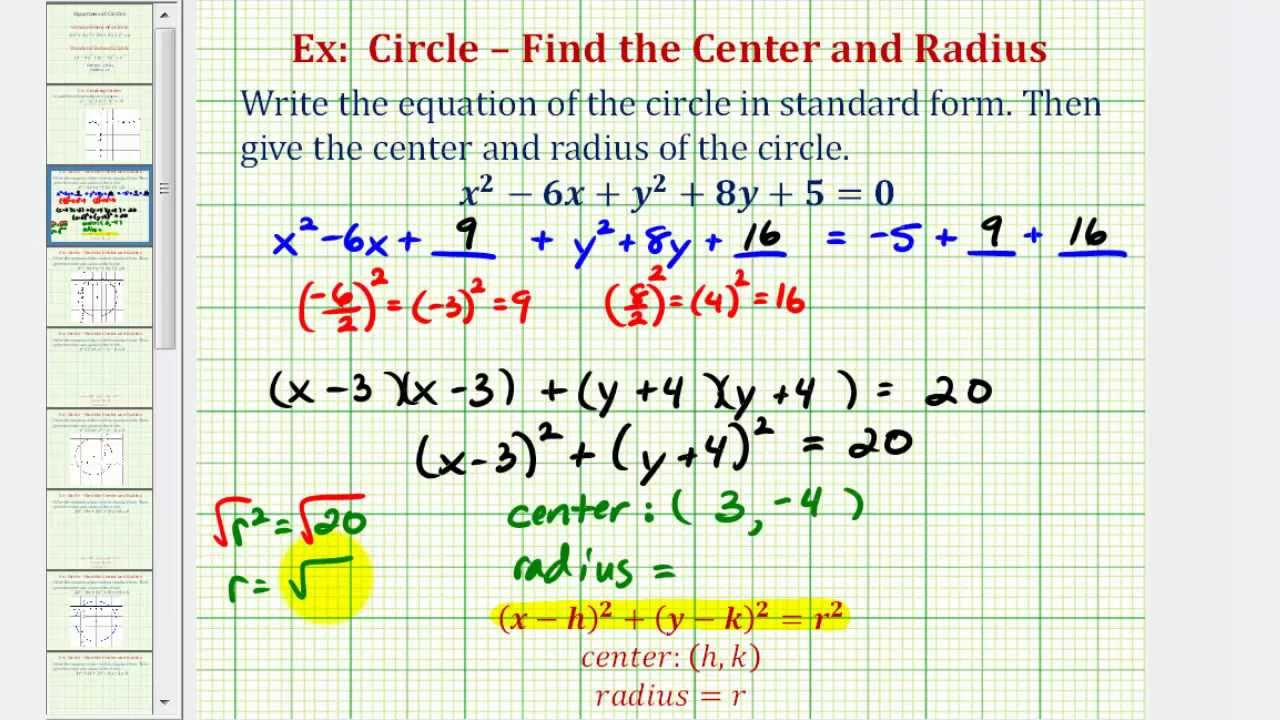
What are its center h k and its radius r. So lets just remind ourselves what a. Find the equation of the circle passing through the points p21 q05 r 12 method 2. Use centre and radius form of the circle.
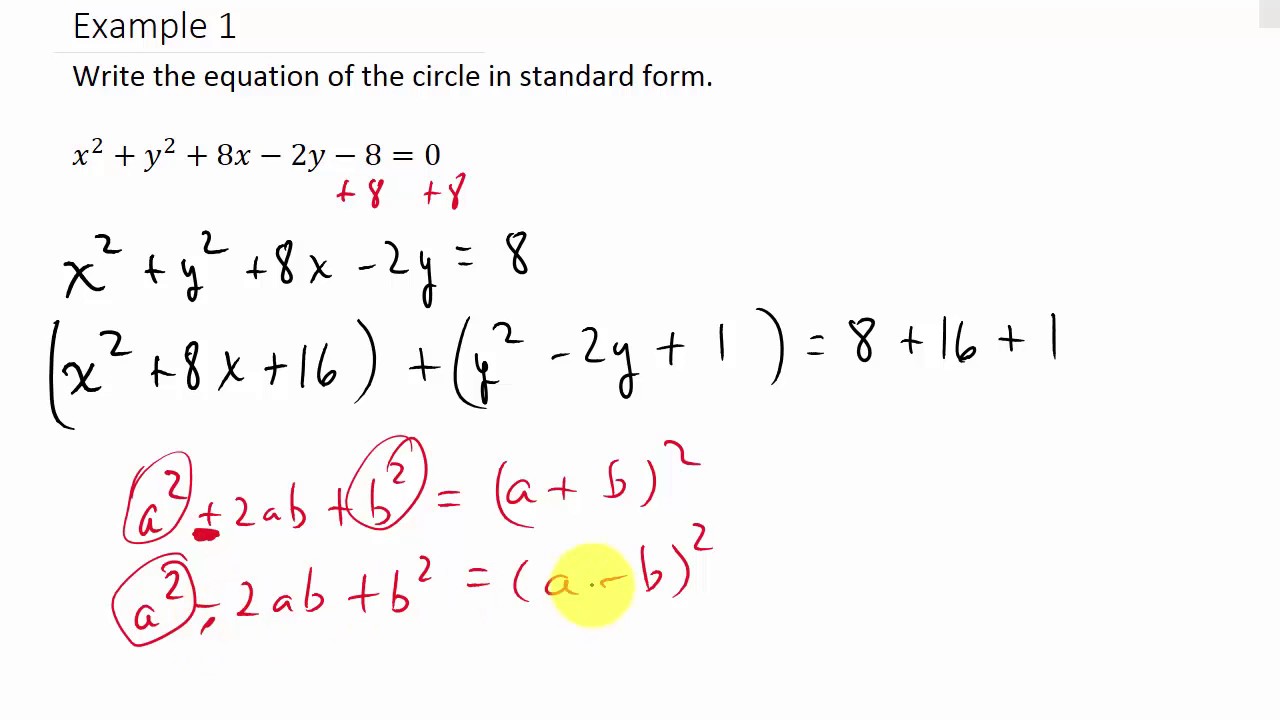
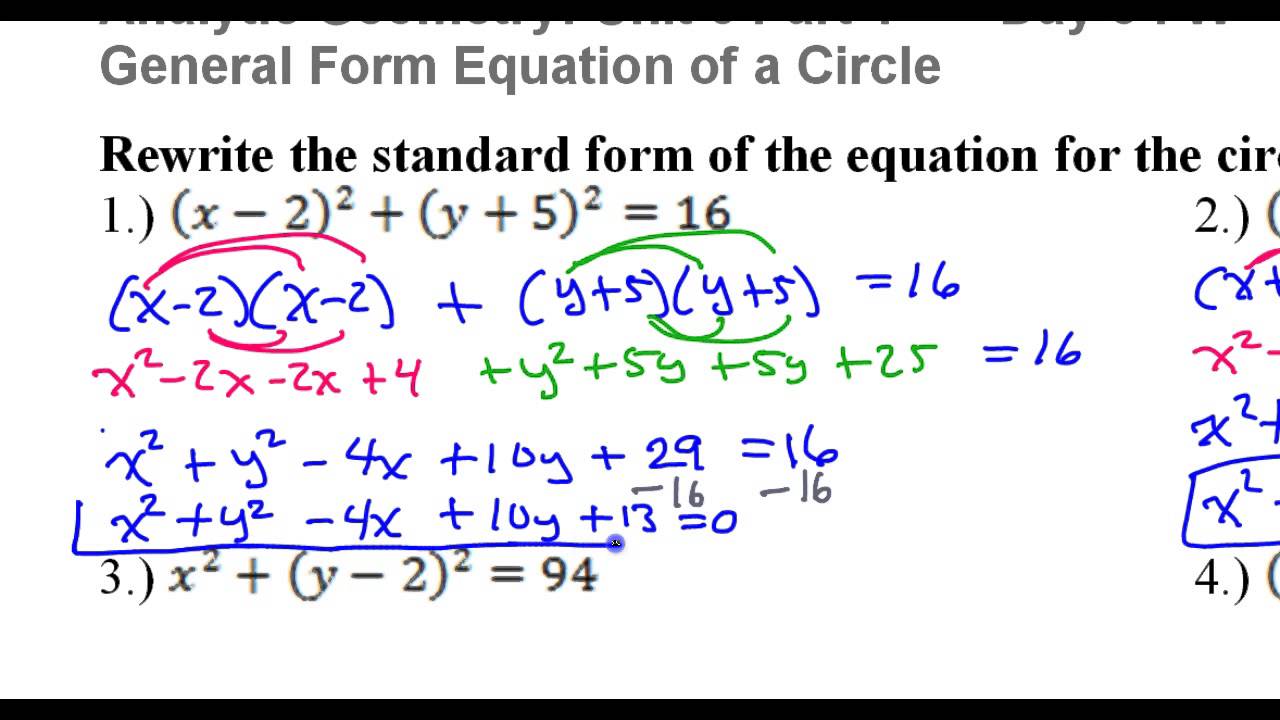
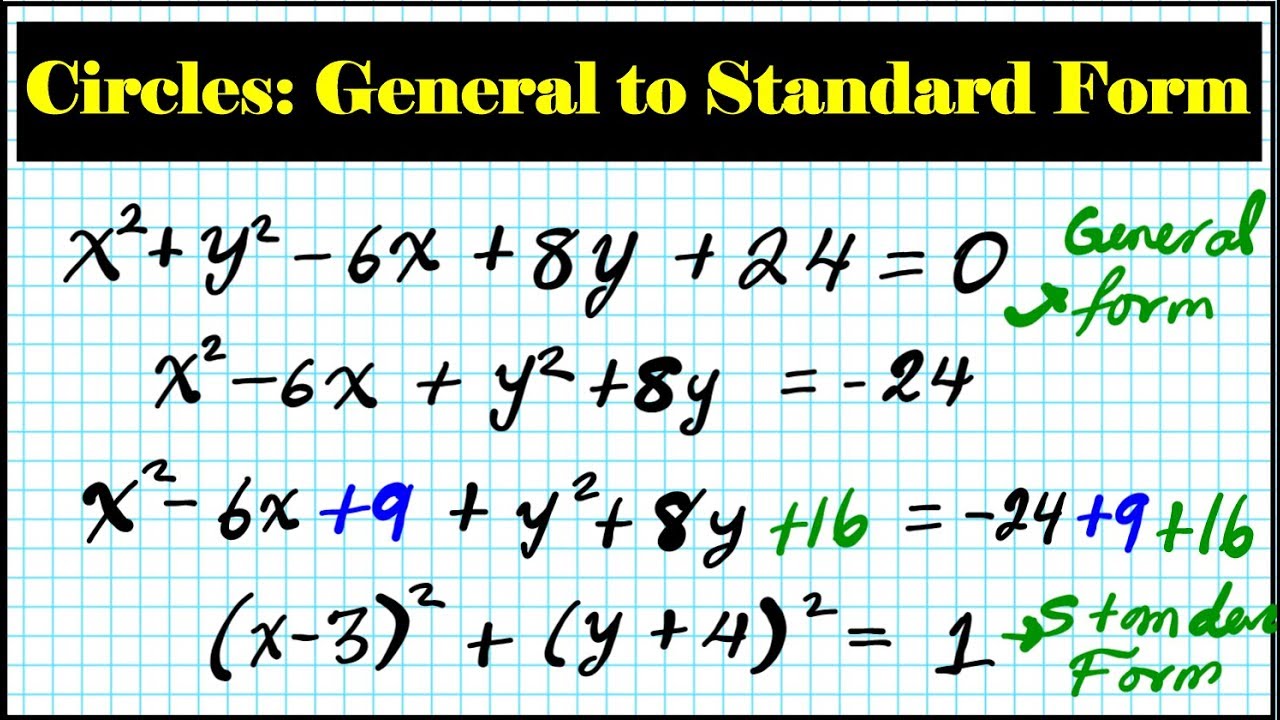
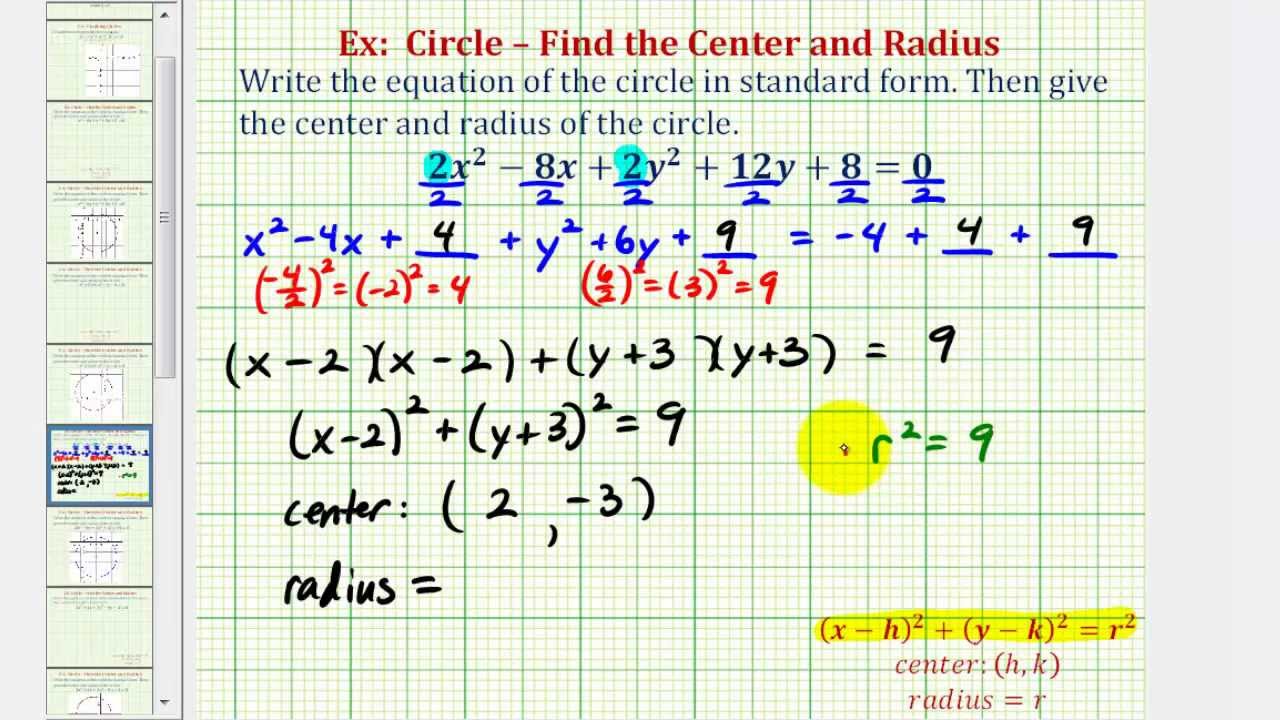
Let the center and radius of. Given a circle on the coordinate plane sal finds its standard equation which is an equation in the form x a2y b2r2. General form always has x 2 y 2 for the first two terms. Going from general form to standard form.
Now imagine we have an equation in general form. If cartesian coordinates are introduced such that the origin is the center of the ellipse and the x axis is the major axis and the foci are the points. A circle can be defined as the locus of all points that satisfy an equation derived from trigonometry.